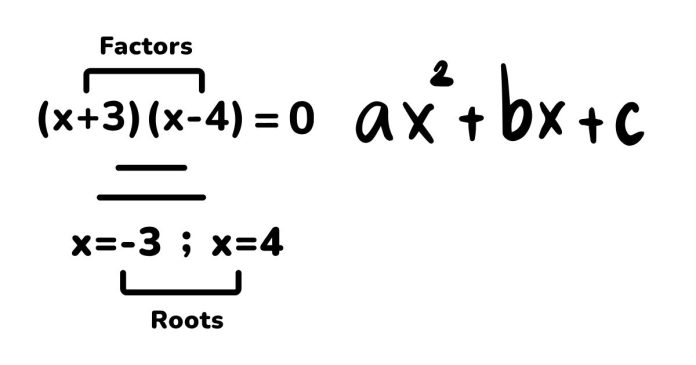Which Of the Following Are Polynomials?

To determine which expressions are polynomials, it’s important to understand the definition of a polynomial. A polynomial is an algebraic expression consisting of terms that are the product of a constant and a non-negative integer exponent of a variable. The general form of a polynomial is:

^an​^xn+^an−^1​xn−1+⋯+a1_​x+a0​

where ^an,^an−1,…, a0 are constants (called coefficients), and the exponents of $x$ are non-negative integers (0, 1, 2, etc.).

Here are some examples to determine whether they are polynomials:

1. 2x²+3x+5 – This is a polynomial because it consists of terms where the exponents of x are non-negative integers (2, 1, and 0).
2. 4x⁻²+7x+3– This is not a polynomial because the term x−2x has a negative exponent, which is not allowed in a polynomial.
3. The square root of x+6 – This is not a polynomial because x sqrt​ can be written asx^1/2, and fractional exponents are not allowed in polynomials.
4. x³$-4x+2$ – This is a polynomial because all exponents are non-negative integers (3, 1, and 0).

So, expressions with only non-negative integer exponents of the variable are polynomials, while others with negative or fractional exponents are not.